
GEOMETRIC SEQUENCE EXAMPLES FREE
You can sign up for a free account here and take a look around at our free resources before you subscribe too. How much did they pay for all 5 books? (Assume that the price of subsequent books continues to rise using the same multiplier).Ġ.65 + 1.95 + 5.85 + 17.55 + 52.65 = £78.65ĭid you like our post on geometric sequences? If so, you can find more of our blogs here! You can also subscribe to Beyond for access to thousands of secondary teaching resources. In this sequence, a is the first term, r is the common ratio found by dividing the subsequent term with preceding term, for example 116/58 232/116 2. The first book cost 65p, the second book cost £1.95 and the third book cost £5.85. How much did they pay for all 5 books? (Assume that the price of subsequent books continues to rise using the same multiplier). Write down the next two terms in each sequence: Write down the multiplier for each sequence.Ģ. This means to find the next term in the sequence, we multiply the last known term by or 0.2:Įither way, the next term in the sequence is 24. So for example, this is a geometric sequence. This means to find the next term in the sequence, we divide the last known term by 5:ĭivide a smaller term by its adjacent larger term: A series, the most conventional use of the word series, means a sum of a sequence. We now have two options:ĭivide a larger term by its adjacent smaller term: Find the terms a 2, a 3, a 4 and a 5 of a geometric sequence if a 1 10 and the common ratio r - 1. In a geometric sequence, a term is determined by multiplying the previous term by the rate, explains to. Now, multiply the last known term in the sequence (3750) by the multiplier (5): We would begin by finding the multiplier: Sometimes, you might be asked to write down the next term(s) in a sequence. On the first leg where it only travels down.Write down the next term in the following sequence: The ball travels this distance in each direction (up and down), except We quickly notice that the terms have a common ratio of 3, so this is the sum of a geometric sequence whose explicit formula is $a_n=5(3^=60$.

Numerical Results for a Partial SumĬonsider the sum $5+15+45+135+\ldots+2657205$. However, sometimes the terms of a geometric sequence will approach zero, and in that case, an sum of an infinite number of terms can be found. Geometric Sequences in REAL Life - Examples and Applications.
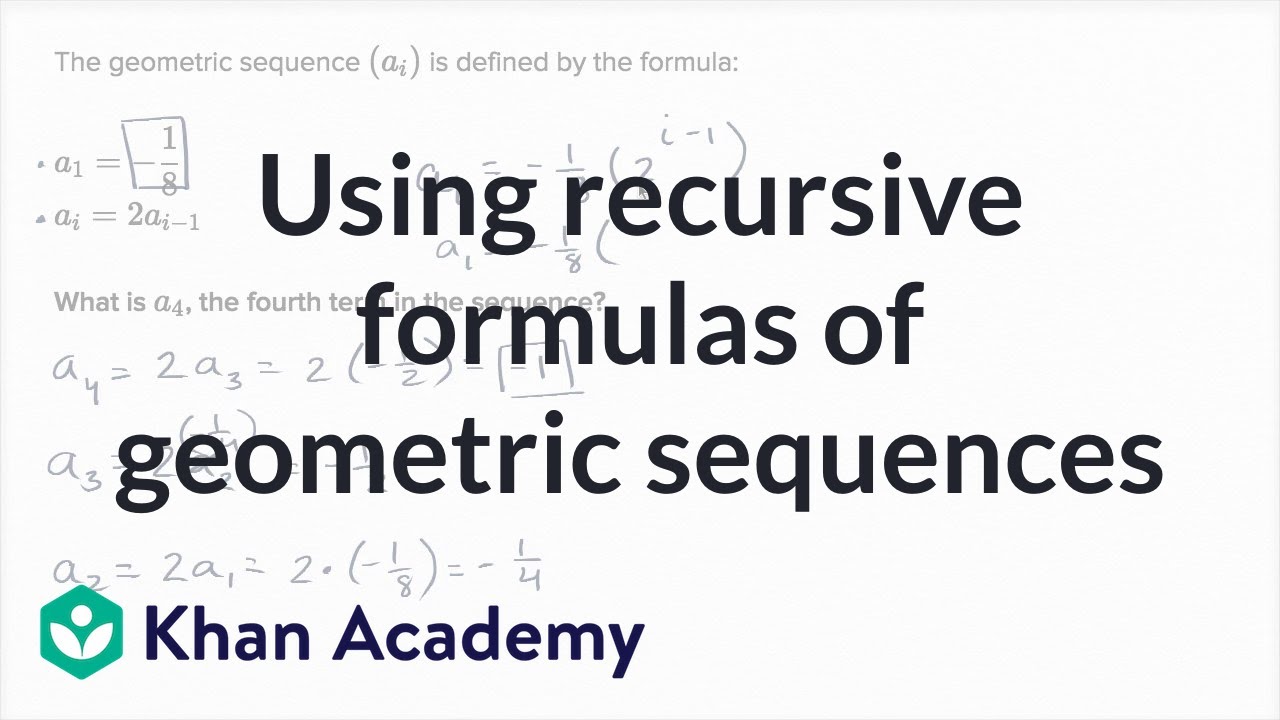
When a geometric sequence has an unbounded long-term behavior, we will be restricted to adding a finite number of terms. Solution EXAMPLE 3 Determine the next term in the geometric sequence: 48, 24, 12, 6. A geometric progression is a sequence in which any element after the first is obtained by multiplying the preceding element by a constant called the common. 10 Examples of the nth term of geometric sequences with answers EXAMPLE 1 Find the 4th term of a geometric sequence where the first term is 5 and the common ratio is 2. Solution EXAMPLE 2 What is the next term in the geometric sequence 3, 15, 75, 375. The terms of a geometric sequence can also be added to find their sum. Geometric sequences Examples with answers EXAMPLE 1 Find the next term in the geometric sequence: 4, 8, 16, 32.
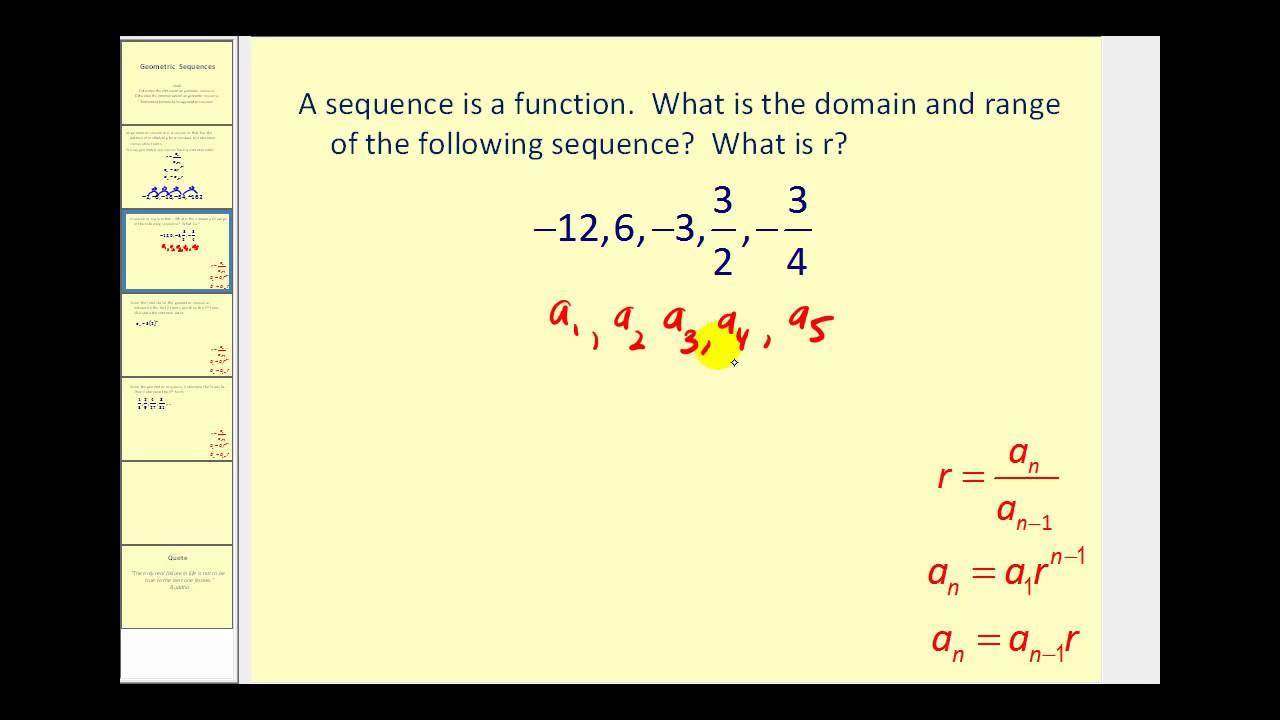
We use MathJax Sums of Geometric Sequences


 0 kommentar(er)
0 kommentar(er)
